|
Books
[1] X. Zhang, Exact
Controllability of Semi-linear Distributed Parameter
Systems, Gaodeng Jiaoyu Chubanshe (Higher
Education Press), Beijing, 2004. (In Chinese). 144
pages. ISBN: 7-04-012953-1.
Invited
Reviews
[1] X. Zhang, Recent progress on
exact controllability theory of the wave and plate
equations, Boletin de la Sociedad Española de
Matemática
Aplicada, 2004, no. 28, 99-128.
Articles published in conference
proceedings
[1] X. Zhang,
Exact internal controllability of Maxwell
equations, Proceedings
of the 17th Chinese Control Conference (1997, Lushan), Wuhan Press,
423-433.
[2] L. Pan, K. L.
Teo and X. Zhang, State
observation problem of a class of semilinear hyperbolic
systems, Proceedings of
The 14th World Congress of IFAC (1999,
Beijing), Vol: E (Robust Control),
Pergamon, 219-224.
[3] X. Zhang,
Observability estimate: a
direct method, Proceedings of the 19th Chinese Control
Conference (2000, Hong Kong), Vol:
1, 181-186.
[4] X. Zhang and E.
Zuazua, The linearized
Benjamin-Bona-Mahony equation: a spectral approach to
unique continuation, Semigroups of Operators: Theory and
Applications(Rio de Janeiro,
2001), C. Kubrusly et
al., eds., 368-379, Optimization
Software, New York, 2002.
[5] X. Zhang and E.
Zuazua, Controllability of
nonlinear partial differential equations, in Lagrangian and
Hamiltonian methods for nonlinear control 2003,
239-243, IFAC,Laxenburg, 2003.
[6] I. Lasiecka, R.
Triggiani and X. Zhang, Nonconservative Schrödinger equations with
unobserved Neumann B. C.: Global uniqueness and
observability in one shot, in Analysis and
Optimization of Differential Systems (Constanta, 2002),
V. Barbu et al., eds.,Kluwer
Acad. Publ., Boston, MA, 2003, 235-246.
[7] X. Zhang,
Global exact controllability of
semi-linear time reversible systems in infinite dimensional
space, Cohen, Gary C. (ed.)
et al., Mathematical and numerical
aspects of wave propagation, WAVES 2003,
Proceedings of the sixth
international conference on mathematical and numerical aspects
of wave propagation, Jyväskylä, Finland, 30, June-4 July 2003,
Berlin, Springer, 183-188 (2003).
[8] X. Zhang and E.
Zuazua, Exact controllability of
the semi-linear wave equation,in Sixty Open Problems in the Mathematics of
Systems and Control, edited
by V. D. Blondel and A. Megretski, Princeton University Press,
2004, 173-178.
[9] X. Zhang,
Some progresses on inverse
hyperbolic problem, Proceedings of
the 22th Chinese Control Conference (2003, Yichang), Wuhan University of Technology
Press, 389-392.
[10] X. Zhang and
E. Zuazua, Stability and control
on a model in fluid-structure interaction,
Proceedings of the
22th
Chinese Control Conference (2003,
Yichang), Wuhan, University of Technology Press, 22-26.
[11] W. Li and X.
Zhang, Controllability of
parabolic and hyperbolic equations: towards a
unified theory, in Control theory of partial differential
equations, Lect. Notes Pure
Appl. Math., 242, Chapman & Hall/CRC, Boca Raton, FL,
2005, 157-174.
[12] J. Yong and X. Zhang, Exact controllability of the heat equation with
hyperbolic memory kernel, in Control theory of
partial differential equations, Lect. Notes Pure Appl.Math., 242, Chapman &
Hall/CRC, Boca Raton, FL, 2005, 387-401.
[13] X. Zhang, Unique continuation for
stochastic partial differential equations and its application,
Boletin de la Sociedad Española de Matematica
Aplicada, 2006, no. 34,
251-256.
[14] X. Fu, X. Zhang and E. Zuazua, On the
optimality of the observability inequalities for plate systems
with potentials, in Phase Space Analysis of PDEs, A. Bove, F.
Colombini, and D. Del Santo, eds., Birkhäuser, 2006, 117-132.
[15] Z. Li and X. Zhang, On fuzzy logic and
chaos theory-from an engineering perspective, Ruan, Wang,
Kerre (Eds.), Fuzzy Logic-A Spectrum of Theoretical &
Practical Issues, Springer-Verlag, 2006.
[16] X. Zhang and E. Zuazua, Asymptotic behavior
of a hyperbolic-parabolic coupled system arising in
fluid-structure interaction, International Series of Numerical
Mathematics, Vol. 154, Birkhäuser, Verlag
Basel/Switzerland, 2006, 445-455.
[17] H. Li and X. Zhang, Periodic
controllability of evolution equations, in Proceedings of the
26th Chinese Control Conference, Vol. 2, Press of
Beihang University, 2007, 651–655.
[17] X. Zhang, Unique continuation and
observability for stochastic parabolic equations and beyond,
Preprint.
[18] X. Zhang, Unique continuation and
observability for stochastic parabolic equations and beyond,
in Control Theory and Related Topics (In Memory of Xunjing
Li), S. Tang and J. Yong, eds., World Sci. Publ.,
Hackensack, NJ, 2007, 147–160.
[19] X. Zhang and E. Zuazua, On the optimality
of the observability inequalities for Kirchoff plate systems
with potentials in unbounded domains, in Hyperbolic Problems:
Theory, Numerics and Applications, S. Benzoni-Gavage and
D. Serre, eds., Springer,2008, 233–243.
[20] X. Zhang, Observability estimates for
stochastic wave equations, in Proceedings of the 27th Chinese
Control Conference, Vol. 3, Press of Beihang
University, 2008, 598–600.
[21] X. Zhang, C. Zheng and E. Zuazua, Exact
controllability of the time discrete wave equation: a
multiplier approach, to appear.
Articles published in
Journal
[1] X. Zhang and F.
Li, Existence, uniqueness
and limit behavior of solutions to a nonlinear boundary-value
problem with equivalued
surface, Nonlinear Anal., 34(1998), no.4, 525-536.
[2] X. Zhang,
Rapid exact controllability of the
semilinear wave equation, Chin. Ann.Math. Ser. B, 20 (1999), no. 3, 377-384.
[3] X. Zhang,
Solvability of nonlinear parabolic
boundary value problem with equivalued surface,
Math. Meth. Appl. Sci.,
22 (1999), no. 3, 259-265.
[4] X. Zhang,
Exact internal controllability of
Maxwell equations, Appl. Math. Optim., 41 (2000), no. 2, 155-170.
[5] X. Zhang,
Explicit
observability estimate for the wave equation with potential
and its application,
Royal Soc. Lond. Proc. Ser. A
Math. Phys. Eng. Sci., 456
(2000), no.1997, 1101-1115.
[6] X. Zhang,
Uniqueness of weak solution for
nonlinear elliptic equations in divergence form,
Internat. J. Math. and Math.
Sci., 23 (2000), no. 5,
313-318.
[7] A. López, X.
Zhang and E. Zuazua, Null controllability of the heat equation as
singular limit of the exact controllability of dissipative
wave equations, J. Math. Pures Appl.,
79(2000), no. 8, 741-808.
[8] I. Lasiecka, R.
Triggiani and X. Zhang, Nonconservative wave equations with unobserved
Neumann B.C.: global
uniqueness and observability in one
shot, in
Differential Geometric Methods in
the Control of Partial Differential Equations,
Contemp. Math.(Amer. Math.
Soc.), 268 (2000),
227-325.
[9] Y. Tang and X.
Zhang, A note on the singular
limit of the exact internal controllability of dissipative
wave equations, Acta
Mathematica Sinica, English Series,
16 (2000), no.4, 601-612.
Shortened Chinese version: 数学学报(英文版),
45 (2002), no. 1,108-116.
[10] X. Zhang,
Exact controllability of semilinear evolution
systems and its applications,
J. Optim. Theory Appl.,
107 (2000), no. 2, 415-432.
[11] L. Li and X.
Zhang, Exact controllability for
semilinear wave equations, J. Math.Anal. Appl., 250 (2000), no. 2, 589-597.
[12] X. Zhang,
Explicit observability inequalities for the wave
equation with lower order terms by means of Carleman
inequalities, SIAM J. Control Optim., 39(2000), no. 3, 812-834.
[13] X. Zhang,
Exact controllability of the semilinear plate
equations, Asymptot. Anal., 27(2001), no. 2, 95-125.
[14] M. Yamamoto
and X. Zhang, Global uniqueness and stability for an inverse
wave source problem with less regular
data,
J. Math. Anal. Appl.,
263 (2001), no. 2, 479-500.
[15] X. Zhang,
A remark on null exact controllability of the
heat equation, SIAM J. Control Optim., 40
(2001), no. 1, 39-53.
[16] K. Liu, B. Rao
and X. Zhang, Stabilization of the
wave equations with potential and indefinite damping,
J. Math. Anal. Appl.,
269 (2002), no. 2, 747-769.
[17] X. Zhang and
E. Zuazua, Unique continuation for the linearized
Benjamin-Bona-Mahony equation with space-dependent
potential,
Mathematische Annalen,
325 (2003),no. 3, 543-582.
[18] X. Zhang and
E. Zuazua, Decay of solutions of the system of
thermoelasticity of type III,
Communications in Contemporary
Mathematics, 5 (2003), no.
1, 25-83.
[19] K. Liu, M.
Yamamoto and X. Zhang, Observability inequalities by internal
observation, J. Optim. Theory
Appl., 116 (2003), no. 3,
621-645.
[20] X. Zhang and
E. Zuazua, Polynomial decay and control of a
1- d
model for
fluid-structure interaction, C. R. Math.
Acad. Sci. Paris, 336
(2003), 745-750.
[21] X. Zhang and
E. Zuazua, Control,
observation and polynomial decay for a coupled heat-wave
system, C. R. Math.
Acad. Sci. Paris, 336 (2003),
823-828.
[22] M. Yamamoto
and X. Zhang, Global uniqueness and stability for a class of
multidimensional inverse hyperbolic problems with two
unknowns, Appl. Math. Optim., 48(2003), no. 3, 211-228.
[23] I. Lasiecka,
R. Triggiani and X. Zhang, Global uniqueness, observability and stabilization of
nonconservative Schrödinger equations via pointwise Carleman
estimates.Part I:
H1(Ω)-estimates, J. Inverse
Ill-Posed Problems, 12
(2004), no. 1, 43-123.
[24] I. Lasiecka,
R. Triggiani and X. Zhang, Global
uniqueness, observability and stabilization of nonconservative
Schrödinger equations via pointwise Carleman estimates.
Part II: L2(Ω)-estimates, J. Inverse
and Ill-Posed Problems,
12 (2004), no. 2, 183-231.
[25] L. Pan, K. L.
Teo and X. Zhang, State-observation problem for a class of
semi-linear hyperbolic systems (Chinese),
Chinese Ann. Math. Ser.
A, 25 (2004), no. 2,
189-198.English version: Chinese Journal of
Contemporary Mathematics, 25 (2004), no. 2, 163-172.
[26] X. Zhang and
E. Zuazua, Polynomial decay and control of a
1-d hyperbolic
-parabolic coupled system, Journal of Differential
Equations,
204 (2004), no. 2, 380-438.
[27] L. C. Wang and
X. Zhang, Inequalities generated
by chains of Jensen inequalities for convex functions,
Kodai Mathematical
Journal, 27 (2004), no. 2,
114-133.
[28] I. Lasiecka,
R. Triggiani and X. Zhang, Carleman estimates at the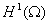 -and
L2(Ω)-level for nonconservative Schrödinger
equations with unbounded Neumann B.C.,
Archives of Inequalities and
Applications, 2 (2004), no.
2 & 3, 215-338. -and
L2(Ω)-level for nonconservative Schrödinger
equations with unbounded Neumann B.C.,
Archives of Inequalities and
Applications, 2 (2004), no.
2 & 3, 215-338.
[29] S. Tang and X.
Zhang, Carleman inequality for backward stochastic
parabolic equations
with general
coeffcients, C. R. Math. Acad. Sci. Paris,
339 (2004), no. 11,
775-780.
[30] J. Rauch, X.
Zhang and E. Zuazua, Polynomial decay of a
hyperbolic-parabolic coupled
system, J.
Math. Pures Appl., 84 (2005), no. 4,
407-470.
[31] B. Guo and X.
Zhang, The regularity of multi-dimensional wave
equation with partial Dirichlet control and
observation, SIAM J. Control
Optim., 44 (2005), no. 5,
1598-1613.
[32] L. Pan, X. Zhang
and Q. Chen, Approximate solutions to infinite dimensional
LQ problems over infinite time horizon, Science in China
Series A-Mathematics, 49 (2006), no. 7, 865-876.
Chinese version: Zhongguo Kexue A Ji-Shuxue, 36 (2006),
no. 5, 588-600.
[33] X. Zhang and E.
Zuazua, A sharp observability inequality for Kirchoff plate
systems with potentials, Comput. Appl. Math., 25
(2006), no. 2-3, 353–373.
[34] L. C. Wang and X.
Zhang, New inequalities related to the Jensen-type
inequalities with repetitive sample, International Journal of Applied Mathematical
Sciences, 3 (2006), no. 1, 51–67.
[35] X. Zhang and E.
Zuazua, Long time behavior of a coupled heat-wave system
arising in fluid-structure interaction, Archive for Rational
Mechanics and Analysis, 184 (2007), no. 1,
49–120.
[36] K. Phung, G. Wang
and X. Zhang, On the existence of time optimal control of
some linear evolution equations, Discrete and Continuous
Dynamical Systems, Series B, 8(2007), no. 4,
925–941.
[37] X. Fu, J. Yong
and X. Zhang, Exact controllability for the
multidimensional semilinear hyperbolic equations, SIAM J.
Control Optim., 46 (2007), no. 5, pp.
1578–1614.
[38] T. Duyckaerts, X.
Zhang and E. Zuazua, On the optimality of the observability
inequalities for parabolic and hyperbolic systems with
potentials, Annales de
l'Institut Henri Poincaré (C) Analyse Non
Linéaire,
25 (2008), no. 1, pp.
1–41.
[39] X. Zhang,
Unique continuation for stochastic parabolic equations,
Differential and
Integral Equations, 21 (2008), no. 1-2 , pp.
81–93.
[40] K. Phung and X. Zhang, Time reversal
focusing of the initial state for Kirchoff plate, SIAM J.
Appl. Math., 68 (2008), no. 6, pp. 1535–1556.
[41] X. Zhang, Carleman and observability
estimates for stochastic wave equations, SIAM J. Math. Anal.,
40(2008) no. 2, pp. 851–868.
[42] X. Zhang, C. Zheng and E. Zuazua, Time
discrete wave equations: boundary observability and control,
Discrete and Continuous Dynamical Systems, 23 (2009),
no.1&2, pp.571-604.
[43] J. Yong and X.
Zhang, Heat equation with memory in anisotropic and
non-homogeneous media, in preparation.
[44] X. Fu, J. Yong
and X. Zhang, Controllability and observability of the heat
equations with hyperbolic memory kernel, in preparation.
[45] S. Tang and X. Zhang, Null
Controllability for forward and backward stochastic parabolic
equations, in preparation.
[46] J. Yong and X.
Zhang, A mixed parabolic and hyperbolic equation with
memory, in preparation.
|